Three Paths on a Tree

Description:

Input:

The first line contains one integer number $$$n$$$ ($$$3 \le n \le 2 \cdot 10^5$$$) — the number of vertices in the tree.

Next $$$n - 1$$$ lines describe the edges of the tree in form $$$a_i, b_i$$$ ($$$1 \le a_i$$$, $$$b_i \le n$$$, $$$a_i \ne b_i$$$). It is guaranteed that given graph is a tree.

Output:

In the first line print one integer $$$res$$$ — the maximum number of edges which belong to at least one of the simple paths between $$$a$$$ and $$$b$$$, $$$b$$$ and $$$c$$$, or $$$a$$$ and $$$c$$$.

In the second line print three integers $$$a, b, c$$$ such that $$$1 \le a, b, c \le n$$$ and $$$a \ne, b \ne c, a \ne c$$$.

If there are several answers, you can print any.

Sample Input:

8
1 2
2 3
3 4
4 5
4 6
3 7
3 8

Sample Output:

5
1 8 6

Note:

The picture corresponding to the first example (and another one correct answer):

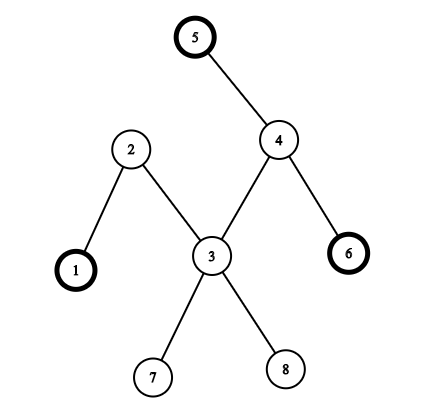
If you choose vertices $$$1, 5, 6$$$ then the path between $$$1$$$ and $$$5$$$ consists of edges $$$(1, 2), (2, 3), (3, 4), (4, 5)$$$, the path between $$$1$$$ and $$$6$$$ consists of edges $$$(1, 2), (2, 3), (3, 4), (4, 6)$$$ and the path between $$$5$$$ and $$$6$$$ consists of edges $$$(4, 5), (4, 6)$$$. The union of these paths is $$$(1, 2), (2, 3), (3, 4), (4, 5), (4, 6)$$$ so the answer is $$$5$$$. It can be shown that there is no better answer.