Four Points

2000ms

262144K

Description:

You are given four different integer points $$$p_1$$$, $$$p_2$$$, $$$p_3$$$ and $$$p_4$$$ on $$$\mathit{XY}$$$ grid.

In one step you can choose one of the points $$$p_i$$$ and move it in one of four directions by one. In other words, if you have chosen point $$$p_i = (x, y)$$$ you can move it to $$$(x, y + 1)$$$, $$$(x, y - 1)$$$, $$$(x + 1, y)$$$ or $$$(x - 1, y)$$$.

Your goal to move points in such a way that they will form a square with sides parallel to $$$\mathit{OX}$$$ and $$$\mathit{OY}$$$ axes (a square with side $$$0$$$ is allowed).

What is the minimum number of steps you need to make such a square?

Input:

The first line contains a single integer $$$t$$$ ($$$1 \le t \le 10^4$$$) — the number of test cases.

Each test case consists of four lines. Each line contains two integers $$$x$$$ and $$$y$$$ ($$$0 \le x, y \le 10^9$$$) — coordinates of one of the points $$$p_i = (x, y)$$$.

All points are different in one test case.

Output:

For each test case, print the single integer — the minimum number of steps to make a square.

Sample Input:

Sample Output:

8
7
5

Note:

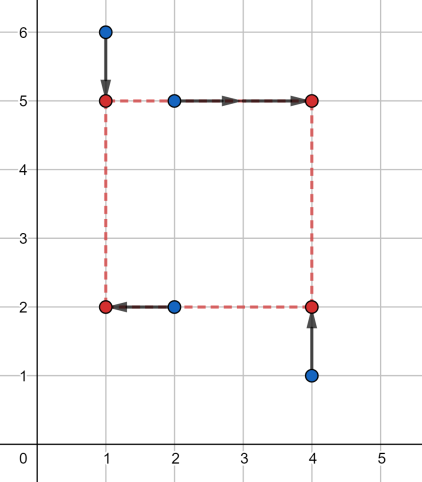
In the first test case, one of the optimal solutions is shown below:

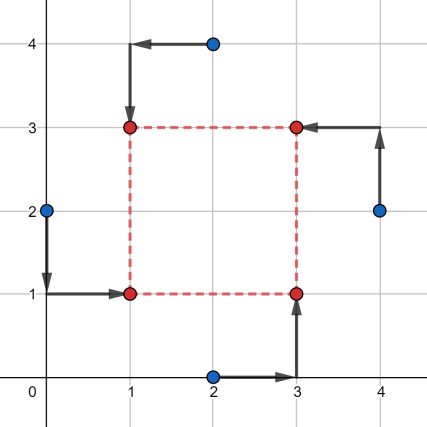
$\text{[math]}$ Each point was moved two times, so the answer $$$2 + 2 + 2 + 2 = 8$$$.

In the second test case, one of the optimal solutions is shown below:

$\text{[math]}$ The answer is $$$3 + 1 + 0 + 3 = 7$$$.

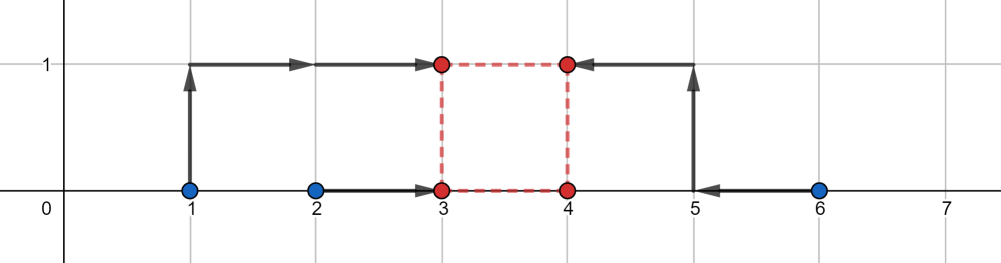
In the third test case, one of the optimal solutions is shown below:

$\text{[math]}$ The answer is $$$1 + 1 + 2 + 1 = 5$$$.

Informação

Provedor Codeforces

Código CF1455E