Colors and Intervals

Description:

Input:

The first line contains two integers $$$n$$$ and $$$k$$$ ($$$1 \le n \le 100$$$, $$$2 \le k \le 100$$$) — the number of colors and the number of occurrences of each color.

The second line contains $$$n \cdot k$$$ integers $$$c_1, \, c_2, \, \dots, \, c_{nk}$$$ ($$$1 \le c_j \le n$$$), where $$$c_j$$$ is the color of number $$$j$$$. It is guaranteed that, for each $$$1 \le i \le n$$$, it holds $$$c_j = i$$$ for exactly $$$k$$$ distinct indices $$$j$$$.

Output:

Output $$$n$$$ lines. The $$$i$$$-th line should contain the two integers $$$a_i$$$ and $$$b_i$$$.

If there are multiple valid choices of the intervals, output any.

Sample Input:

4 3
2 4 3 1 1 4 2 3 2 1 3 4

Sample Output:

4 5
1 7
8 11
6 12

Sample Input:

1 2
1 1

Sample Output:

1 2

Sample Input:

3 3
3 1 2 3 2 1 2 1 3

Sample Output:

6 8
3 7
1 4

Sample Input:

2 3
2 1 1 1 2 2

Sample Output:

2 3
5 6

Note:

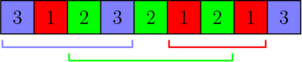
In the first sample, each number can be contained in at most $$$\left\lceil \frac{4}{3 - 1} \right\rceil = 2$$$ intervals. The output is described by the following picture:

In the second sample, the only interval to be chosen is forced to be $$$[1, \, 2]$$$, and each number is indeed contained in at most $$$\left\lceil \frac{1}{2 - 1} \right\rceil = 1$$$ interval.

In the third sample, each number can be contained in at most $$$\left\lceil \frac{3}{3 - 1} \right\rceil = 2$$$ intervals. The output is described by the following picture: