Keen Tree Calculation

Description:

Input:

The first line contains a single integer $$$N$$$ ($$$2\leq N\leq10^5$$$) — the number of vertices in the tree.

The $$$i$$$-th of the next $$$N-1$$$ lines contains three integers $$$U_i$$$, $$$V_i$$$, and $$$W_i$$$ ($$$1 \leq U_i,V_i \leq N$$$; $$$1\leq W_i\leq10^9$$$) — an edge that connects vertices $$$U_i$$$ and $$$V_i$$$ with a length of $$$W_i$$$. The edges form a tree.

The $$$(N+1)$$$-th line contains a single integer $$$Q$$$ ($$$1\leq Q\leq10^5$$$) — the number of questions.

The $$$j$$$-th of the next $$$Q$$$ lines contains two integers $$$X_j$$$ and $$$K_j$$$ as described ($$$1 \leq X_j \leq N$$$; $$$1 \leq K_j \leq 10^9$$$).

Output:

Output $$$Q$$$ lines with an integer in each line. The integer in the $$$j$$$-th line represents the diameter of the tree on the $$$j$$$-th question.

Sample Input:

7
5 1 2
1 4 2
3 4 1
2 5 3
6 1 6
4 7 2
2
4 3
3 2

Sample Output:

18
11

Sample Input:

3
1 2 1000000000
2 3 1000000000
1
2 1000000000

Sample Output:

2000000000000000000

Note:

In the first example, the following is the tree without any changes.

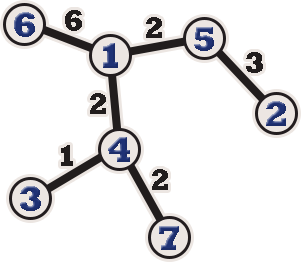
The following is the tree on the $$$1$$$-st question.

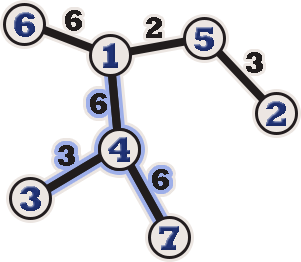
The maximum distance is between vertices $$$6$$$ and $$$7$$$, which is $$$6+6+6=18$$$, so the diameter is $$$18$$$.

The following is the tree on the $$$2$$$-nd question.

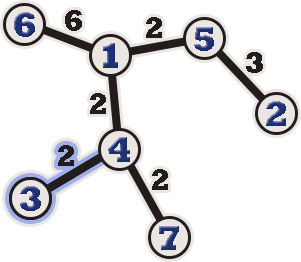
The maximum distance is between vertices $$$2$$$ and $$$6$$$, which is $$$3+2+6=11$$$, so the diameter is $$$11$$$.